
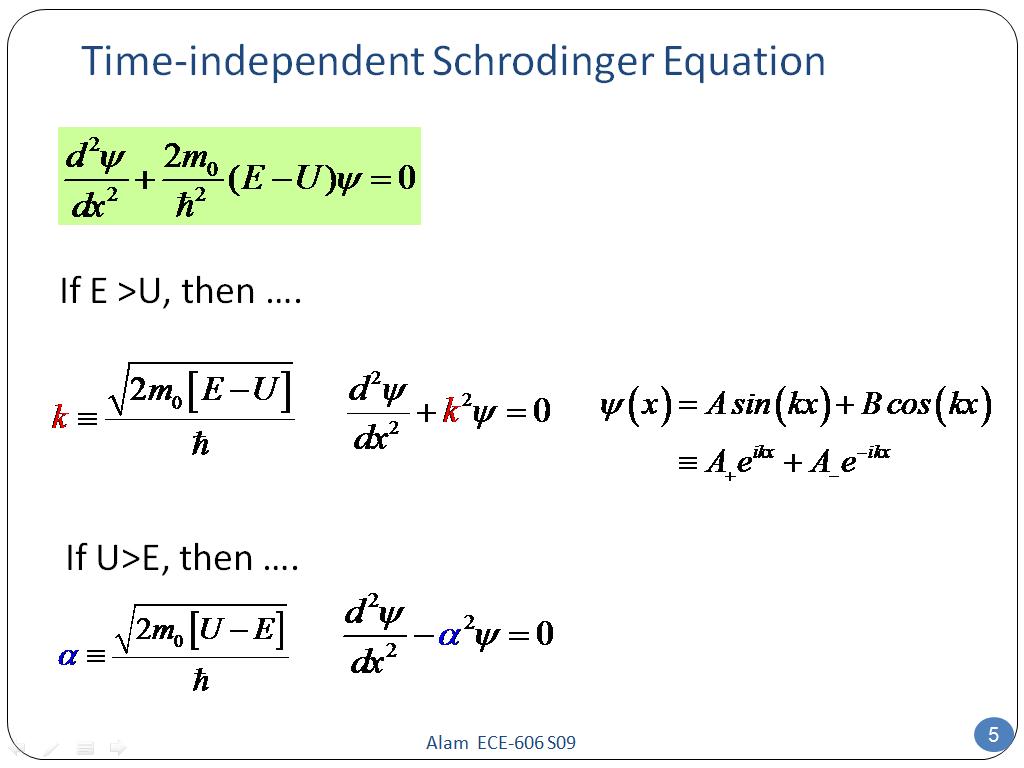
Now we consider the general case, where $n$ and $m$ are not necessarily equal, and the system can be degenerate. El Proyecto ESVI-AL procura mejorar la accesibilidad de la educación superior virtual.
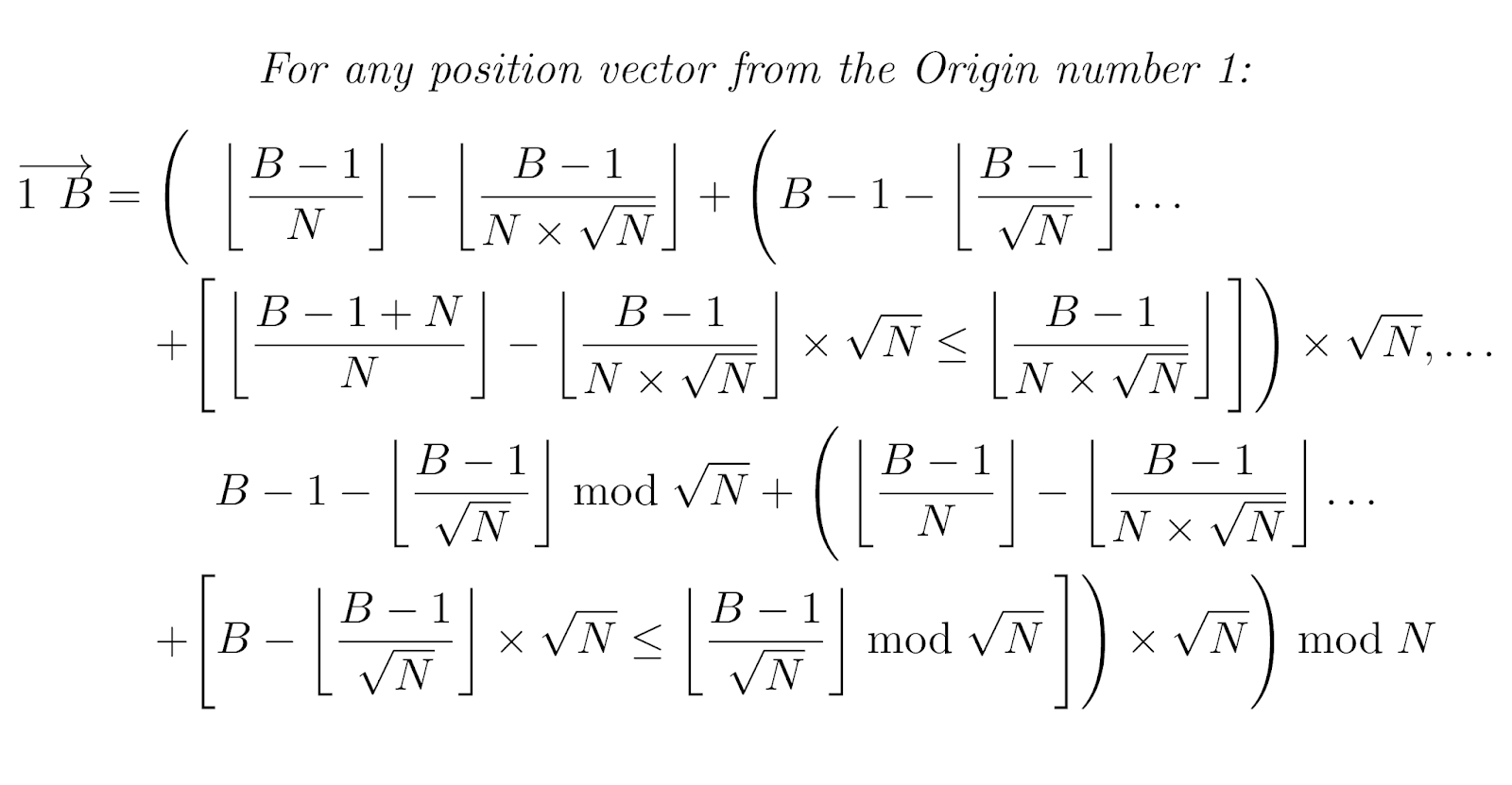
it has non-zero determinant, and has unique solution), the algorithm described above will transform $A$ into identity matrix. In the case where $m = n$ and the system is non-degenerate (i.e. Without this heuristic, even for matrices of size about $20$, the error will be too big and can cause overflow for floating points data types of C++. This heuristic is used to reduce the value range of the matrix in later steps. The Stern-Brocot Tree and Farey SequencesĪ_$. Optimal schedule of jobs given their deadlines and durationsġ5 Puzzle Game: Existence Of The Solution MEX task (Minimal Excluded element in an array) Search the subsegment with the maximum/minimum sum RMQ task (Range Minimum Query - the smallest element in an interval) Kuhn's Algorithm - Maximum Bipartite Matching Maximum flow - Push-relabel algorithm improved Maximum flow - Ford-Fulkerson and Edmonds-Karp
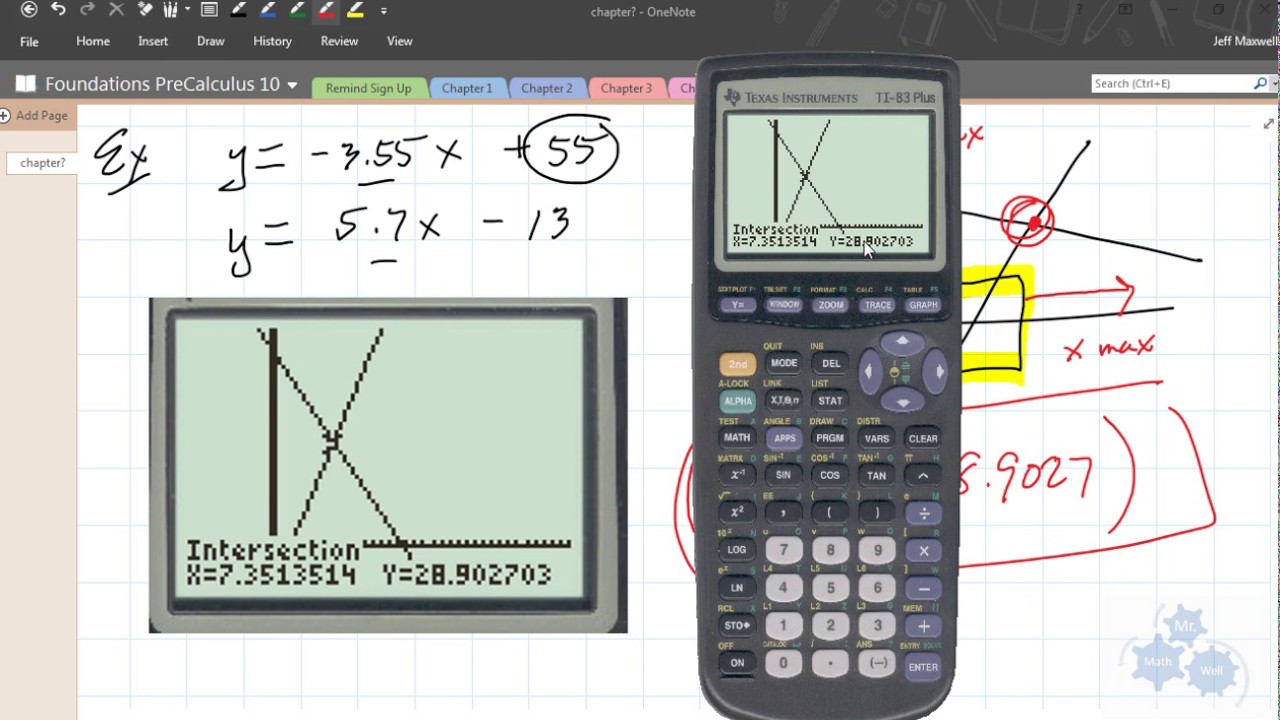
If you use this method, then it doesn’t matter how each equation is set up. Mei Li, Curtis Clement, Satvik Golechha, and contributed. Lowest Common Ancestor - Tarjan's off-line algorithm When solving linear systems, you have two methods at your disposal, and which one you choose depends on the problem: If the coefficient of any variable is 1, which means you can easily solve for it in terms of the other variable, then substitution is a very good bet. Lowest Common Ancestor - Farach-Colton and Bender algorithm Second best Minimum Spanning Tree - Using Kruskal and Lowest Common AncestorĬhecking a graph for acyclicity and finding a cycle in O(M) Minimum Spanning Tree - Kruskal with Disjoint Set Union Number of paths of fixed length / Shortest paths of fixed length Strongly Connected Components and Condensation Graphĭijkstra - finding shortest paths from given vertexīellman-Ford - finding shortest paths with negative weightsįloyd-Warshall - finding all shortest paths Half-plane intersection - S&I Algorithm in O(N log N)Ĭonnected components, bridges, articulations points Search for a pair of intersecting segmentsĭelaunay triangulation and Voronoi diagram Pick's Theorem - area of lattice polygons Manacher's Algorithm - Finding all sub-palindromes in O(N)Ī little note about different heuristics of choosing pivoting rowīurnside's lemma / Pólya enumeration theoremįinding the equation of a line for a segmentĬheck if points belong to the convex polygon in O(log N) Euclidean algorithm for computing the greatest common divisorĭeleting from a data structure in O(T(n) log n)ĭynamic Programming on Broken Profile.


 0 kommentar(er)
0 kommentar(er)
